1. Does the function
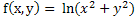
satisfy Laplace’s equation?
Yes, a direct calculation shows
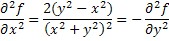
2.Find an expression for the potential in the region between two infinite parallel planes 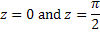 on which the potentials are respectively given by
on which the potentials are respectively given by 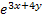 and 0.
and 0.
In this case also we have solutions which are written as products of separate variables. The solution can be written as (since X and Y are hyperbolic functions, the function Z is a linear combination of sine and cosine),
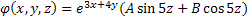
The constants A and B can be determined by the boundary conditions. For z=0, 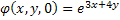 gives B=1. For
gives B=1. For 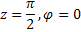 gives A=0. Thus
gives A=0. Thus
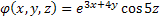
3. Find an expression for the potential in the region between two infinite parallel planes, the potential on which are given by the following :
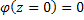
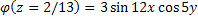
![]() on which the potentials are respectively given by
on which the potentials are respectively given by ![]() and 0.
and 0. ![]()
![]()